A normal shaped price-yield curve, such as the one given below, suggests that a bond’s price may not increase by the same absolute amount when interest rates fall by a certain % as when prices decrease due to an interest rate rise by that same %. This is because of the curvature or convexity of the yield curve.
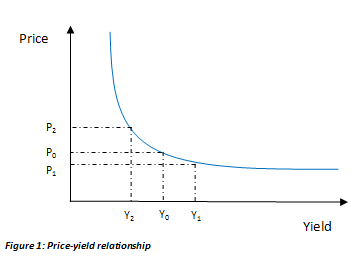
The absolute changes in yields Y1-Y0 and Y2-Y0 are the same yet the price increase P2-P0 is greater than the price decrease P1-P0.
When interest rates increase, prices fall, but for a bond with a more convex price-yield curve that fall is less than for a bond with a price-yield curve having less curvature or convexity. When interest rates decline the bonds price increases. Again for a bond whose price-yield curve has greater convexity the price increase is more than for a bond whose price-yield relation is less convex by comparison. This is illustrated below:
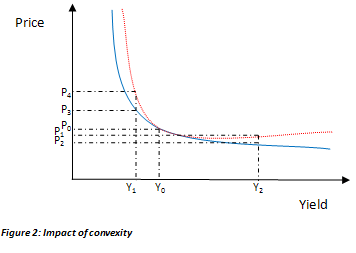
When Yields increase from Y0 to Y2 the price of the bond with the more convex price yield relationship (red curve) decreases by a smaller amount (from P0 to P1) than the price of a bond with the less convex price yield relationship (blue) (P0 to P2). When yields decrease from Y0 to Y1, the price of the bond with the more convex yield curve shows a much higher increase (from P0 to P4) as opposed to the price of the bond with a less convex price-yield relation (from P0 to P3).
The Duration measure approximates the change in the price of a bond, but that estimate is the same regardless of when the interest rates increase or decrease. This means that the duration measure ignores the shape or curve of the price/ yield function, in fact modified duration is a linear approximation to the actual price-yield relationship. While it does provide a sufficiently accurate approximation of the price change for small parallel shifts in the yield curve, it is a poor estimate of price change for larger parallel shifts. In such instances the convexity approximation, the second price approximation function, improves on the price change estimate. This is demonstrated in the example below:
The total estimated percentage change in price is given by the following equation:
Approximate price change (%)= -Duration×Delta_i×100+Convexity×(Delta_i)2×100
where Delta_i is the yield change.
Let us assume that we have a 10% semi-annual coupon bearing bond maturing in 1 year. The bond has a face amount of 100. The duration and convexity measures of the bond given the yield changes by 2% from 12% to 14% (or 12% to 10%) are 0.9209 and 0.6462 respectively.
For an increase in yields
When the yield increases from 12% to 14% the price of the bond decreases from 98.1666 to 96.3840. The actual price change is -1.8159%.
Using only duration the estimated price change works out to:
(-0.9209*0.02)% = -1.8418%
Whereas using duration and convexity the estimated price change is:
-1.8418% + (0.6462*0.02^2)% = -1.8159%, i.e. it equals the actual price change.
In the same way, if yields were to decrease by 2% from 12% to 10% the price of the bond increases from 98.16666 to 100. The actual price change is 1.8676%.
The estimate using duration only remains unchanged in absolute terms from that given above. There is only a sign change, i.e. the estimated price change is 1.8418%.
Whereas using duration and convexity the estimated price change is:
1.8418% + (0.6462*0.02^2)% = 1.8676%, i.e. is equals the actual price change.
Therefore including the convexity measure adjusts the duration only approximated price change in such a way as to result in a better estimation of actual price change.
Modified, Effective, Positive, Negative
The terms modified and effective in relation to both duration and convexity are used based on whether or not the expected future cash flows of the underlying instrument are assumed to change or not when yields change. If the cash flows are assumed to remain unchanged when yields change the term modified is used. Alternatively if the cash flows are assumed to change in response to changes in the interest rates the term effective is associated with both the duration and convexity measures.
Because of this assumption of whether cash flows will change or not, the results from the calculations of modified convexity and effective convexity can be very different. In the case of option free bonds where the cash flows would not vary if interest rates were to vary, the convexity measure, regardless of whether modified or effective, would always be positive.
Positive convexity means that a price fall occurs at a slower rate when interest rates increase by a certain percentage as compared to the price rise when increase rates decrease by that same percentage. Alternatively negative convexity means than a price fall occurs at a faster rate when interest rates increase by a certain percentage as compared to the price rise when interest rates fall by that same percentage (i.e. price-yield curve function is concave).
In the case of bonds with options, where the future cash flows are not certain and could change when yields changes, the results obtained from the calculation of modified and effective convexity could be very different sometimes resulting in negative effective convexity while displaying positive modified convexity.
Convexity in Asset Liability Management
In order to immunize a portfolio against interest rates changes, a portfolio manager would like the assets and liabilities to respond in an identical manner when interest rates change. This is why a portfolio manager will try to match the duration of assets to the duration of liabilities in order to make the assets as sensitive to interest rate changes as the liabilities response to these changes.
However the calculation of duration implies a parallel shift in the yield curve and a linear relationship between price and yield. In the real world interest rate changes and price yield relationships do not conform to these underlying assumptions of duration. So an additional constraint is added which would ensure that the present value of assets would increase by more (or decrease by less) than the present value of liabilities when interest rates change. The additional constraint that would make this possible is ensuring that the convexity of assets is greater than the convexity of liabilities.
If you are interested in practical application of duration and convexity in Asset Liability Management reporting and systems see ALM for Board Members – 7 lessons in 60 minutes.
