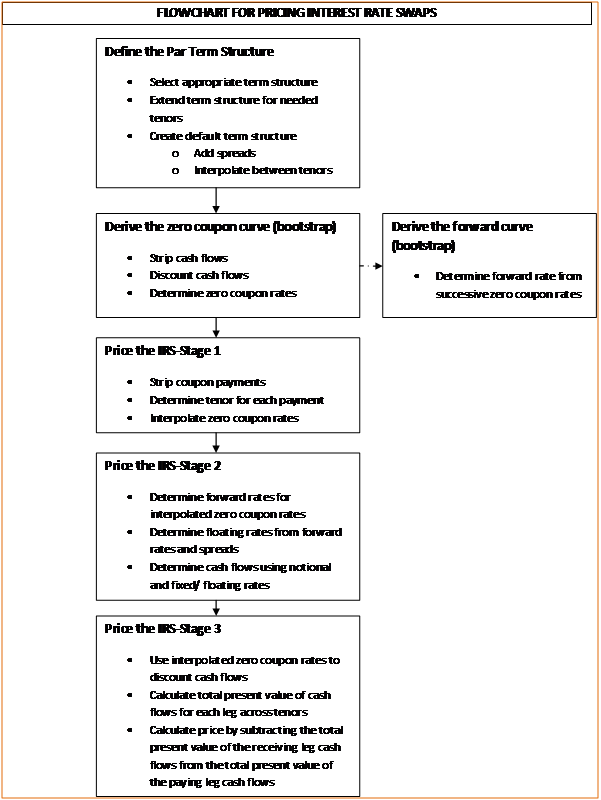
The following process will be followed when determining the value or price of an interest rate swap. Firstly, a default par term structure will be defined. This consists of selecting an appropriate par term structure based on the terms of the interest rate swap, in particular the coupon rate payments and the frequency of the payments. As given par structures may only be available for specified tenors, if the tenors required for the swap exceed the maximum tenor available in the chosen term structure, rates from another term structure may be chosen. A spread may be added to these rates to reflect the differential between these two par term structures. For cases where the payment frequency is semiannual or quarterly, etc, par rates may not be available for all tenors. These rates will have to be determined by interpolating the available rates.
After the par term structure has been determined, the zero coupon rates and are derived using the bootstrapping methodology. This consists of stripping each coupon bearing instrument represented in the par term structure into individual cash flows, iteratively working through each tenor of the par term structure starting with the smallest, discounting cash flows, substituting the derived discount rates to determined the entire zero curve. The zero curve is then used to derive forward rates for each successive interest rate period, again using a bootstrapping methodology.
Once we have the zero curve we are ready to start with the pricing of the interest rate swaps. We strip each coupon from the structure and determine the time to maturity or tenor for each from the date of valuation to the date when the payment is due. Using the calculated tenors and the zero coupon rates derived earlier, interpolated zero coupon rates for the specific IRS instrument being priced will be determined. These interpolated zero coupon rates will be used to a) discount the cash flows and b) derive the forward rates which will serve as the basis for the future coupon rates of the floating leg (s) of the transaction.
Forward rates will be calculated by applying the bootstrapping formula to the interpolated zero coupon rates to determine the rate applicable to each successive interest rate period. The floating rate will be the forward rate so determined plus a spread if applicable.
Cash flows for the fixed payment leg will be simply the fixed rate times the notional amount. For the floating leg the cash flows will be the forward rate (plus spread) times the notional amount. These cash flows will be discounted using the interpolated zero rates over the term between the due date and the pricing date. The total present values will be sum of these discount cash flows and the price of the instrument is the difference between the total present values of the receiving leg and the paying leg.
The flow chart below summarizes the process. This is then followed by a detailed step-by-step write up on the process together with numerical examples.

As an analyst at FNB and 3rd year Bcomm in Banking Management student, i fing your website more helpful then some of the assistance given to me by my assigned tutors! Thanks! 🙂