Earlier we had defined the input and calculation cells for the Heath-Jarrow-Merton (HJM) multifactor no-arbitrage model in EXCEL. In this post, we will see how the output cells, i.e. the price matrix, path prices and true prices, are constructed.
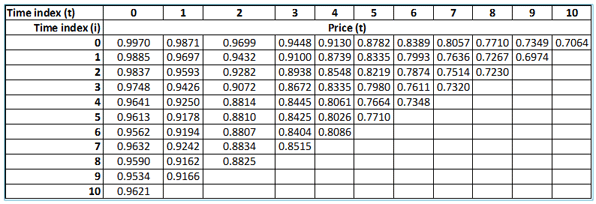 |
| Standing at the origin, (Time Index (i) as given by the first column) the prices at each future node/ time step, t are calculated as follows:Price0(t)=Exp(-?Ratej.dt) where the summation is from j=0 to t and Rate0 is the respective spot rate where as Ratej for j › 0 are the respective Forward Rates determined in the previous step for Time Index i=0 (as given by the first column above).For example Price0(0) is the price of a zero coupon bond at time 0 maturing for 1 one period later. Price0(1) is the price of zero coupon bond at time 0 that will maturing for 1 two periods later and so on.The same process is applied to determine prices when standing at time index i=1,2,…,10 (as given by the first column above. For example, Price1(0) is the price of a zero coupon bond at time 1 maturing for 1 one period later. |
Path Prices and True Prices |
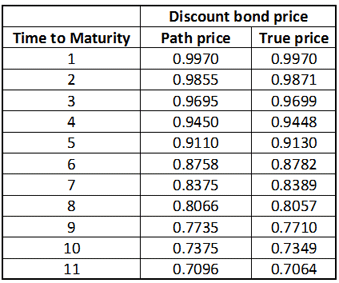 |
| At origin, the Path Prices for each future path is derived by the multiplication of successive Pricet(0), i.e. Path Price (t) = . For example the Path Price at origin for a zero coupon bond with 2 years to maturity is Price0(0)* Price1(0) = 0.9970*0.9885 = 0.9855.The True Prices are the prices derived using the forwards rates implicit in the initial zero rate curve as given by the first row of rates in the spot and forward rates derived earlier. For example the True Price at origin for a zero coupon bond with 2 years to maturity is:Exp(-(0.30%+1%)*1) = 0.9871Comparison of the two price series is given in the graph below: |
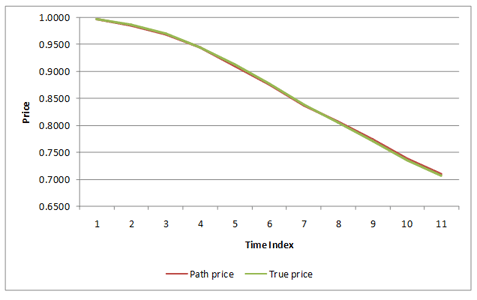 |
| In this post we reviewed how the output cells are defined for the HJM model. This primarily involves a price matrix as well as a table comparing the Path Prices for a zero coupon instrument determined from the model to the True Prices observed at the current point in time (i.e. the results of the calibration process).In the final set of posts to the interest rate simulation crash course we will be reviewing the Principal Component Analysis (PCA) process. This is the process that is employed to determine the volatility functions or factors which are some of the main inputs to an HJM interest rate model and to calibrate the model to observed data.If you would like to buy this course as a PDF file or the sample EXCEL sheets, please see the Interest Rate Modelling section at our online finance course store. |
