Convexity
Duration approximates the change in price of an instrument due to changes in the yield. However this approximation tends to work only for small changes in yield. For larger changes there will be a significant error term between the actual price change and that estimated using duration. Convexity improves on this approximation as it explains the change in price that Duration does not explain.
The formula for measuring Convexity is as follows:
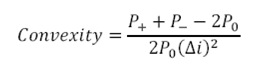
Where,
Δi= change in yield (in decimals)
P0= Initial Price
P_plus (P+) = Price if yields increase by Δi
P_minus (P–) = Price if yields decline by Δi
Another important aspect of the convexity approximation is that it takes into account the curvature of the price/ yield relationship. The Duration measure assumes the size of approximated change in price (in percentage terms) are the same if yields were either to increase or decrease by the same amount. Convexity, depending on the sign, accounts for the direction of the change in yield and the curvature of the price/ yield relationship. A positive convexity measure indicates a greater price increase when interest rates fall by a given percentage relative to the price decline if interest rates were to rise by that same percentage. A negative convexity measure indicates that the price decline will be greater than the price gain for the same percentage change in yield. This feature of convexity plays an interesting role in Asset Liability Management calculations and in contracts that have embedded options.
Approximate price change
The approximate price change using both the duration and convexity measures will be as follows:
Total estimated percentage price change= -Duration×Δi×100+Convexity×(Δi)2×100
Terminology: Modified and Effective
Use the term modified with duration and convexity when we assume that the changes in yield do not to affect the expected cash flows of the security (e.g. for option free instruments). Use the term effective with duration and convexity when we assume that the changes in yield also impact the expected cash flows of the security (e.g. for derivatives).
Please also see:

Comments are closed.