For path dependent and forward starting options it is important to assess Vega, the sensitivity of the option’s value to changes in volatility, and in particular to assess these sensitivities for forward buckets. The first step in this process is to determine how to calculate forward volatilities for these forward buckets from spot volatilities implied in current market option prices.
The procedure for determining these volatilities is similar to the procedure used for determining forward rates from spot prices.
Similarities with bootstrapping the forward rate curve
Given the spot rates for a zero coupon security maturing at time 1 and a zero coupon security maturing at time 2, (where time 1 < time 2), it is possible through bootstrapping, to calculate the forward rate for the period between time 1 and time 2.
Similarly given the spot volatilities for the period t0 to t1 (σt0, t1) and t0 to t2 (σt0, t2) respectively, it is possible to infer the expected volatility between t1 and t2 (σt1, t2). This is the forward implied volatility (also known as the forward-forward volatility) for the period [t1, t2].
Taleb’s Formula for annualized forward implied volatilities
In ‘Dynamic Hedging’ Nicholas Nassim Taleb presents the formula for computing the annualized forward implied volatility for the period between [tn-α, tn], , as follows:
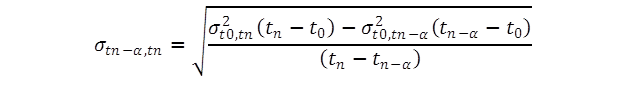
Where:

This formula accounts for unequal nonoverlapping time steps in line with how spot implied volatilities and options prices are quoted in the market.
Step 1 – Obtain option volatility data
For example, let us consider the following annualized spot volatilities for at-the-money call options of strike 272 on Barclay’s stock (BARC) for 31-Jan-2014 obtained from ivolatility.com.
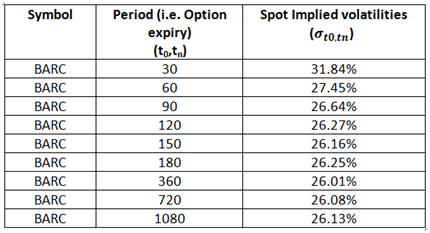
Where t0 is time 0.
Step 2 – Calculate the annualized spot variances
Using the data provided first calculate the annualized variance for the periods (t0,tn), i.e.
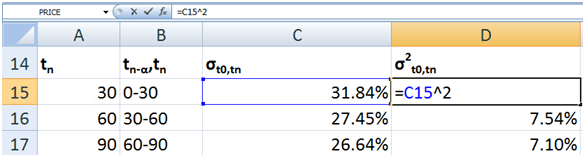
Step 3 – Calculate the annualized forward variances
Then calculate the annualized forward variance for the period [tn-α, tn], σ2tn-α,tn. For the first period 0-30, the forward variance will be the same as the spot variance. For later periods, 30-60, 60-90, 90-120, …, 360-720 & 720-1080, the forward variance will be calculated using Taleb’s formula as follows:
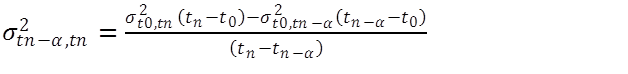
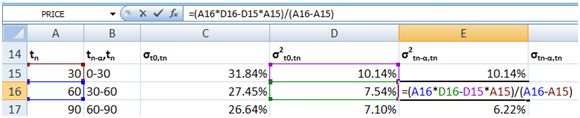
Step 4 – Determine the annualized forward implied volatilities
The square root of this forward variance will be the annualized forward volatility for the period [tn-α, tn]:
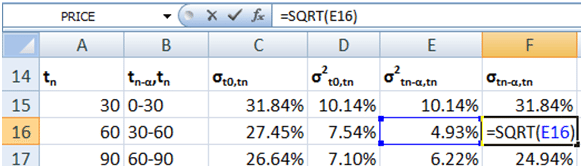
For the entire data set the forward volatilities are as follows:
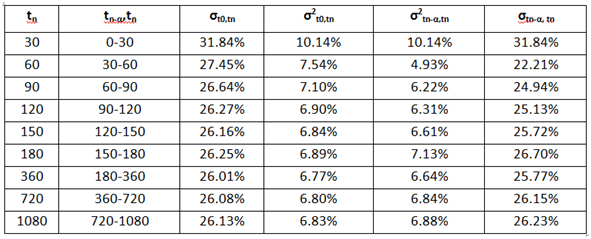
The graphical plot of the spot and forward volatilities is given below:
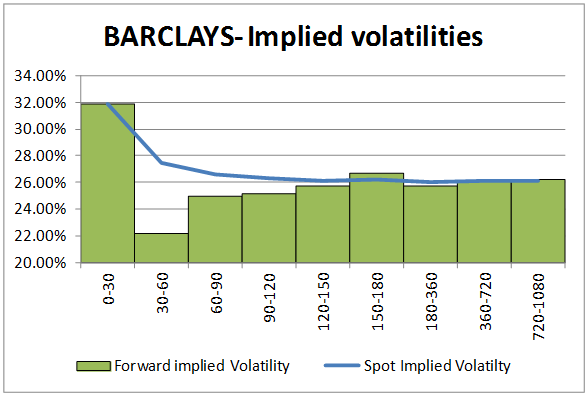
Forward implied volatility between two points is the ‘local volatility’ between (S, t) and (S, t+Δt). The generalization of this formula gives Dupire-Derman-Kani’s local volatility which is a function of time to expiry and option moneyness.
Comparing local, implied and forward volatilities
Since we have spent a fair bit of time with NVDA options in earlier posts while charting the local volatility surface for NVDA, it would be instructive to see how the forward implied volatility for NVDA option plots when we apply Taleb’s implied forward volatility formula.
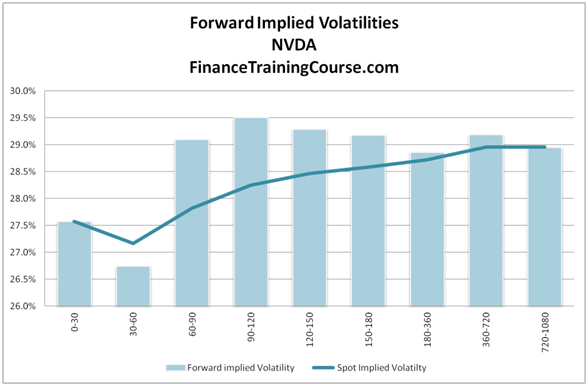
The forward volatilities will change for every series of option expiries for a given strike price.
It is also useful to compare all three volatilities (implied, local and forward) on the same grid for the same series of options.
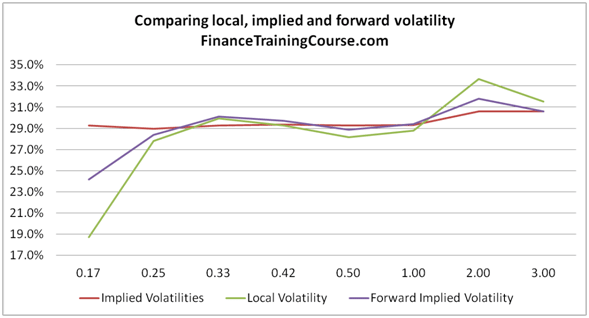
We would have expected to see a convergence between local and forward volatilities and the plot above shows some tracking between these two numbers. The raw spot volatility data is relatively flat when compared to the other two estimates.
